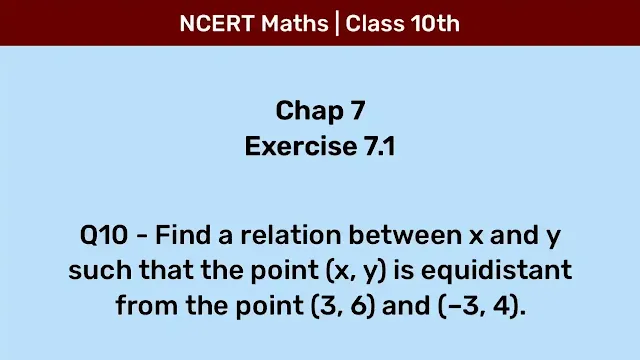
Ex 7.1 Q10 - Find a relation between x and y such that the point (x, y) is equidistant from the point (3, 6) and (– 3, 4).
Solution:-Let, given points be A(x, y) , B(3, 6) and C(-3, 4)
➙ Since the point (x, y) is equidistant from the points (3, 6) and (-3, 4)
∴ AB = AC
∴ AB² = AC²
*Using distance formula
D² = (x₂-x₁)² + (y₂-y₁)²
∴ (x - 3)² + (y - 6)² = (x + 3)² + (y - 4)²
∴ x² - 6x + 9 + y² - 12y +36 = x² + 6x + 9 + y² - 8y + 16
∴ x² + y² - 6x - 12y + 45 = x² + y² + 6x -8y + 25
∴ -6x - 12y + 45 = 6x - 8y + 25
∴ 6x + 6x - 8y + 12y = 45 - 25
∴ 12x + 4y = 20
*Taking 4 as common
∴ 3x + y = 5
∴ 3x + y - 5 = 0
Hence,
The relation between x and y such that the point (x, y) is equidistant from the point (3, 6) and (– 3, 4) is 3x + y - 5 = 0.
------------------------------------
Try This..
✒ Find the value of x for which the distance from (x, 5) to (1, –2) is 10 units.
✒ Show that the point (2, –1) lies on the perpendicular bisector of the line segment joining (3, 6) and (–3, 4).
✒ Find a relation between x and y such that the point (x, y) is equidistant from (4, –3) and (–2, 5).
❌ Common Mistakes
Forgetting to square both sides:▸ Always remove square roots by squaring both sides, a small mistake can lead to a completely wrong equation.Wrong expansion:▸ Watch out while expanding squares like . Don’t forget the middle term .Skipping simplification:▸ Students often leave the final equation without simplifying – aim for the neatest form!Cancelling incorrectly:▸ Only cancel like terms, especially on both sides of an equation. Double-check your signs!
📝 Related Questions:-
Queries Solved:-
Class 10 Ex 7.1
Ex 7.1 Q10 Class 10
Class 10 Ex 7.1 Q10
Class 10 Chap 7 Ex 7.1 Q10
Class 10 Coordinate Geometry
If you found it helpful, please leave a comment below sharing your thoughts or questions.
Don’t forget to share it with your classmates to help them learn too.
Good luck, and happy learning!
Together, let’s make math simpler and more enjoyable for everyone!